Research Interests

Nanoscaled structures such as carbon nanotubes and fullerenes which are not bonded together undergo interactions described as van der Waals forces. At very small scales these interactions can lead to extreme accelerations, velocities and, in the case of oscillating systems, frequencies. By modelling the structures as surfaces with uniform atomic densities and the van der Waals interactions using a 6-12 Lennard-Jones potential, we can make predictions regarding these systems including deriving a formula for the frequency which is in good agreement with molecular dynamics simulations.

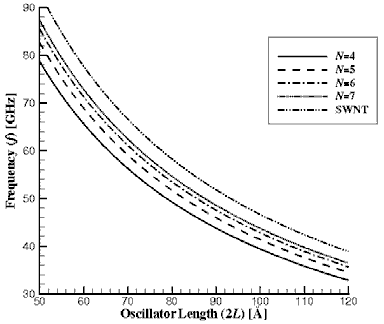
Frequency for a fullerene nanotube bundle oscillator for various configurations and for a fullerene oscillating in a single-walled carbon nanotube

It is clear from the various structures seen at the nanoscale such as the the cylindrical shapes of nanotubes and spherical and spheroidal shapes of fullerenes that the complex interactions of these structures often lead to symmetric conformations. So in satisfying a minimum energy constraint the system often (although not always) adopts a symmetric structure which shares the energetic costs of bending and stretching covalent bonds equally to all components in the structure. By assuming a symmetric conformation up front, it is possible to reduce fundamentally complex problems of molecular structure to problems with are more mathematically tractable and thereby derive results which can be confirmed by experiment and simulation and can also be used to predict ideal systems and novel structures in certain extreme cases.

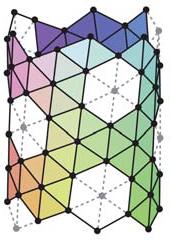
Polyhedral model for a chiral (9,6) boron nanotubes with 1/9 hexagonal holes

In the late 1940s Winslow described a new phenomenon where a fluid-like material would change to become a solid-like gel under the application of an electric field. This phenomenon became known as the Winslow or electrorheological (ER) effect and those materials developed to take advantage of it are referred to as ER fluids. ER fluids comprise small particles dispersed in a non-conducting fluid medium, typically an oil. The size of the particles used can vary, but with the advent of improved processing, miniaturisation and the nanotechnology revolution, ER fluids are now being produced using dispersed particles that have a nanoscale length dimension. One of the central issues with ER fluids has been the relative weakness of the effect under low and moderate electric field strength. Typically, the shear strength that ER fluids have achieved has been less than 100 kPa which has been a major limiting factor in their widespread incorporation in commercial devices. However, recent new ER fluids have been developed with coated nanoscale particles that have achieved yield stresses in excess of 250 kPa.

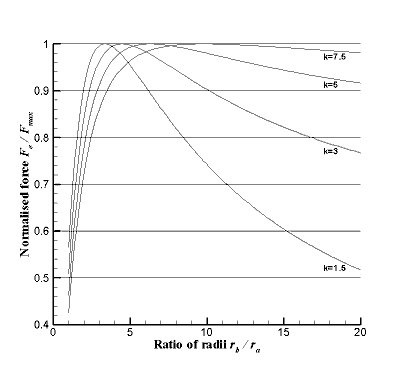
Comparison of mutual force with touching spheres varying the dielectric constant (k) and the ratio of particle radii. The force has been normalised using the maximum value reached for each value of k

Despite the small length scales involved, classical continuum mechanics can still be useful in nanoscale systems. One example of this is in the calculation of intermolecular interaction forces between carbon nanotubes. Carbon nanotubes typically comprise thousands of atoms and performing a rigourous pairwise calculation for several carbon nanotubes can be computationally intensive. By smearing the atoms over the surface of a cylinder, the interaction can be calculated to reasonable accuracy using surface integrals of the potential function over each of two interacting molecules. For certain orientations and choice of potential function, these surface integrals can be expressed as analytical functions such as hypergeometric functions and their relatives

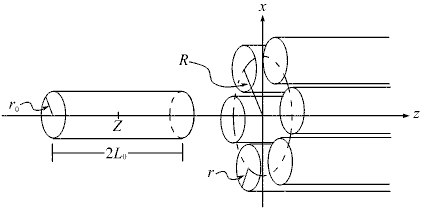
Geometry for a single nanotube entering a nanotube bundle

The design of many novel electronic devices will hinge on our understanding of the joining of certain nanostructures. For example, the joining of a carbon nanotubes to graphene sheets, fullerenes and other carbon nanotubes applies to the situation of constructing nano-electro-mechanical devices including nanoscale FETs. Connecting carbon nanostructures essentially involves a discrete geometric procedure and we have attempted to solve such problems by invoking the principle that the bond lengths and bond angles at the join are determined in such a manner that their their total squared deviation from some ideal configuration is a minimum. We have also applied the calculus of variations to compute various join configurations in such a way that the total curvature squared is minimised, subject to other constraints regarding the interatomic bond lengths.

Relationship between parameters when joining two fullerenes (nanobuds)